Tight binding model on square lattice with stagger flux
Landau guage
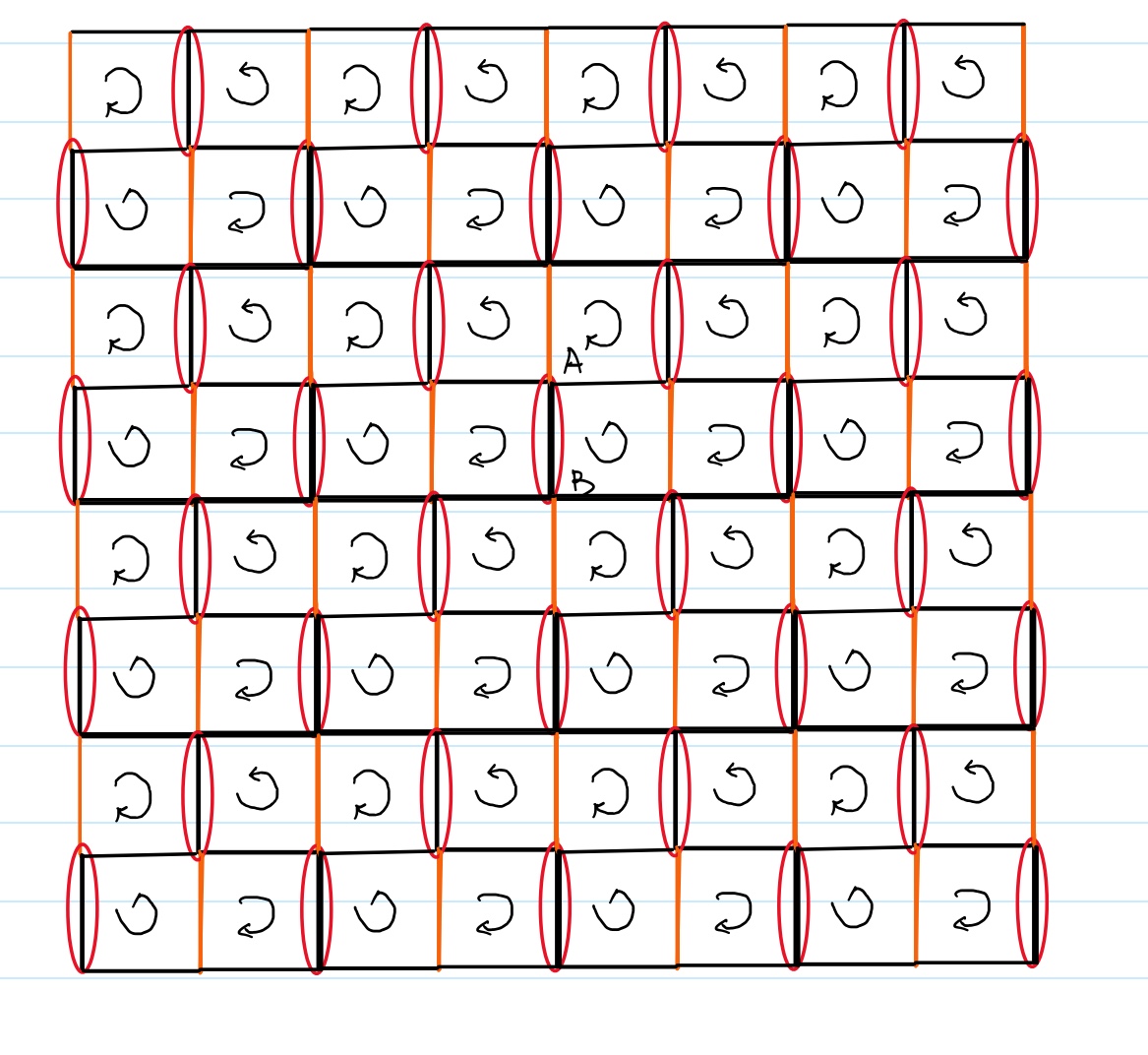
We adopt tranverse gauge to study this problem . The unit cell could be selected as Fig(\@ref(fig:1)) . Every cell has two freedom $A,B$ . The Bloch hamiltonian on the momentum space could be written as
\[\begin{align} H(\mathbf{k}) t=\sum\limits_{\mathbf{k}} \begin{pmatrix} \psi_{A}^{\dagger}(k)& \psi_{B}^{\dagger}(k) \end{pmatrix} \begin{pmatrix} 0 & f(k) \\ f^{*}(k) &0 \end{pmatrix} \begin{pmatrix} \psi_A(k) \\ \psi_B(k)\\ \end{pmatrix} \end{align}\]where $f(k)$ is
\[\begin{align} f(k)=1+e^{\text{i} (k_x-k_y)} +e^{\text{i}(-k_x-k_y)} +e^{-\text{i}(2k_y+\phi)} \end{align}\]The enenrgy spectrum is derived as
\[\begin{align} \varepsilon(k)=\pm \sqrt{ \cos^2k_x +\cos^2(k_y+\frac{\phi}{2}) +2\cos\frac{\phi}{2} \cos k_x \cos(k_y+\frac{\phi}{2}) } (\#eq:3) \end{align}\]We can find the Dirac points of dispersion (\@ref(eq:3)) easily, namly
\[\begin{align} \left( \pm \frac{\pi}{2},\pm \frac{\pi}{2}-\frac{\phi}{2} \right) \quad \left( \mp \frac{\pi}{2},\pm \frac{\pi}{2}-\frac{\phi}{2} \right) \end{align}\]We will give the linear dispersion on symmetric gauge cases.
Symmetric gauge
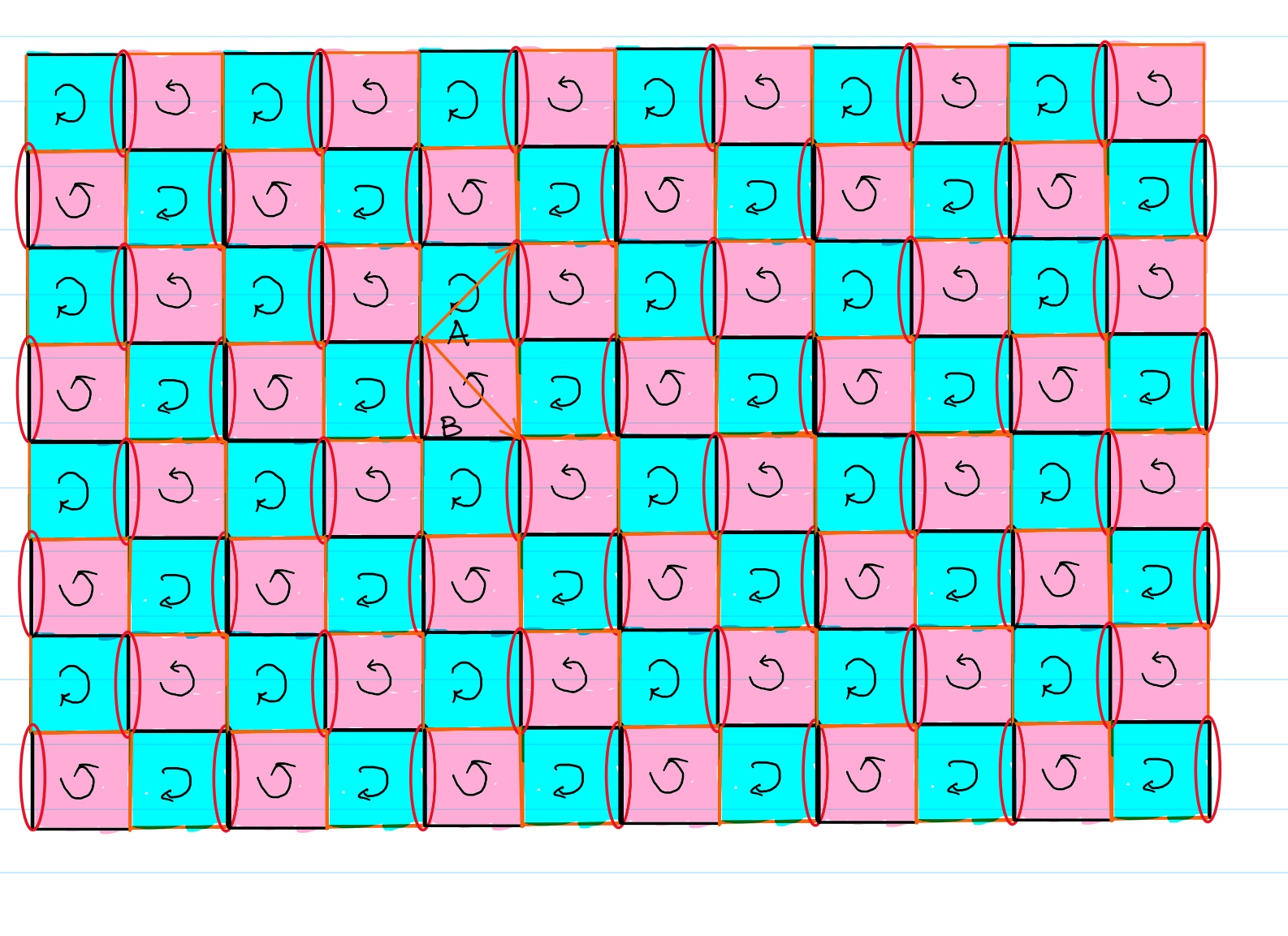
We consider tight binding model on square lattice with stagger flux $\phi$. As shown on Fig(\@ref(fig:2)), the unit cell are selected as the plaquette with positive flux $\phi$. Every unit cell has two freedom $A,B$, the Bloch hamiltonian could be described with four component spinor $\displaystyle \mathbf{\psi}(k)= (\psi_{A}(k)\quad \psi_{B}(k) )^{\mathbf{T}}$ . The Bloch hamiltonian reads as
\[\begin{align} H(\mathbf{k}) t=\sum\limits_{\mathbf{k}} \begin{pmatrix} \psi_{A}^{\dagger}(k)& \psi_{B}^{\dagger}(k) \end{pmatrix} \begin{pmatrix} 0 & f(k) \\ f^{*}(k) &0 \end{pmatrix} \begin{pmatrix} \psi_A(k) \\ \psi_B(k)\\ \end{pmatrix} \end{align}\]where $f(k)$ is
\[\begin{align} f(k)= e^{\text{i} \frac{\phi}{4}} +e^{\text{i}\left( \frac{\phi}{4} -2k_y\right) } +e^{\text{i}\left( k_x-k_y-\frac{\phi}{4} \right) } +e^{\text{i}\left( -k_x-k_y-\frac{\phi}{4} \right) } \end{align}\]Hence, the enenrgy spectrum is just $\varepsilon(k)=\pm \mid f(k)\mid$
\[\begin{align} \varepsilon(k)=\pm 2\sqrt{\cos^2 k_x+\cos^2k_y+2\cos \frac{\phi}{2} \cos k_x\cos k_y} (\#eq:14) \end{align}\]The dispersion relation (\@ref(eq:14)) shows the zero point at $(\pm \frac{\pi}{2},\pm \frac{\pi}{2}), (\mp \frac{\pi}{2},\pm \frac{\pi}{2})$ . We expand the linear dispersion relation at this points.
\[\begin{align} f(k) \mid _{(\frac{\pi}{2},\frac{\pi}{2})}&=t e^{\text{i} \frac{\phi}{4}} \left( 1- e^{-\text{i}(2k_y-\pi)} \right) +e^{-\text{i} \frac{\phi}{4}} \left( e^{\text{i}(k_x-k_y)} - e^{-\text{i}(k_x+k_y-\pi)} \right) \nonumber \\ & \approx 2t\text{i} e^{\text{i} \frac{\phi}{4}} (k_y-\frac{\pi}{2}) + 2\text{i}e^{-\text{i} \frac{\phi}{4}} (k_x-\frac{\pi}{2}) \nonumber \\ &=2t\left( (k_x-k_y)\sin \frac{\phi}{4} +\text{i}(k_x+k_y-\pi) \cos\frac{\phi}{4} \right) \end{align}\]The low enenrgy physics could be described by
\[\begin{align} H(k)=2t \vec{\text{K}} \cdot \vec{\sigma} \qquad \vec{K}=\vec{k} -(\frac{\pi}{2},\frac{\pi}{2}) \end{align}\]